For this part, we were to find the height of the cannon ball and how long it was in the air by using the formula: h = -16t2+v0t+h0. The H represents the final height, the -16 represents the pull of gravity, v0t represents the initial velocity, and h0 represents the initial height.
We were given a problem: “A cannonball is shot upward from the upper deck of a fort with an initial velocity of 192 feet per second. The deck is 32 feet above the ground.”
1.Finding the final height
1st. plug in our know units into the formula :
h=-16t2+192t+32
2nd. Find the value of t. t represents time, use the formula t=-b/(2a). plug in the units from the above equation.
3rd. t=(-192)/(2*-16) simply solve.
4th. t= 6 seconds
5th. then after you have the time, you simply insert it back into the original equation in step 1.
h=-16(6)2+192(6)+32 simply solve once more.
h=-576+1152+32
h=608 feet.
This number represents the vertex of the cannonball in mid air. It is the highest point. This graph is similar to what would occur from a cannon shot. The vertex would be the maximum of the parabola (the round tip), in real life, it would be how high it reaches before gravity pulls it down.
r= range in legthThis number represents the vertex of the cannonball in mid air. It is the highest point. This graph is similar to what would occur from a cannon shot. The vertex would be the maximum of the parabola (the round tip), in real life, it would be how high it reaches before gravity pulls it down.
2. Find the time of how long the cannonball is in the air
This process is definitely more time consuming.
1st. plug in the known units into the formula:
h=-16t2+192t+32
2nd. Create a list of our terms:(this step is not necessary but it just helps organize the information)
a= -16
b= 192
c= 32
3rd. plug all these units properly into the quadratic formula which is:
-b ±√b2-4ac Plug in our know units: -192±√(192)2-4(-16)(32)
2a 2 (-16)
4th. solve what is inside the square root symbol and you should get this:
-192±√38912
-32
5th. do the square root of what is inside the square root symbol:
-192 ± 197.3
-32
6th. divide -32 into the top numbers and you should get this:
6 ± -6.17
t=12.17 seconds
Imagine that the line on the graph is the trail of the cannonball shot in the air. The ball can only last 12.17 seconds in the air
how high did the cannonball go? 608 ft.
how long is the cannonball in the air? 12.17 sec.
~*~*~*~*~*~*~*~*~*~*~*~*~*~*~*~*~
Imagine that the line on the graph is the trail of the cannonball shot in the air. The ball can only last 12.17 seconds in the air
how high did the cannonball go? 608 ft.
how long is the cannonball in the air? 12.17 sec.
~*~*~*~*~*~*~*~*~*~*~*~*~*~*~*~*~
It looks like this formula could be useful in solving for the initial velocity:
g= acceleration of gravity (meter/second^2)
θ= projection angle
however, this seems simpler: (speed (ft/sec))cos(launch angle)
I predict the trajectory will be smooth and the cannonball will shoot straight forward

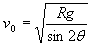
Great job! I love how you included a graph that shoes the parabola and everything. You made the math really easy to understand with your steps.
ReplyDeleteI really like how you included the graph that actually shows the parabola that was graphed! You were able to make it seem like a quick and simple calculation. Overall you did a great job! Also I'm not your "little sister"
ReplyDeletethank you :D
ReplyDeleteProbably one of the best math parts I've seen, including mine. I feel like you explained it really well. The graphics really helped.
ReplyDelete